Getting your Trinity Audio player ready...

Hold down the T key for 3 seconds to activate the audio accessibility mode, at which point you can click the K key to pause and resume audio. Useful for the Check Your Understanding and See Answers.

Fundamentals

The volume of a sample of gas is dependent upon the Kelvin temperature of the gas. Increasing the Kelvin temperature increases the volume. The two quantities are directly proportional to one another. A quadrupling of the Kelvin temperature will quadruple the volum of the gas.

There are two questions in this Question Group. Each question is very similar to one another. The question below is one of the questions.
 

Version 1:

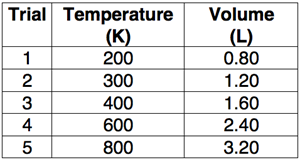Observe the data table shown at the right for a sample of gas that has a constant pressure and number of particles.  Use the data table to answer the next two questions. 
When the Kelvin temperature of the gas is quadruple (increased by a factor of four), the volume of the gas becomes _____.
a. four times larger                                                  b. eight times larger
c. sixteen times larger                                             d. one-fourth the size
e. one-eighth the size                                              f. one-sixteenth the size
g. Not possible to tell
 
Which pairs of trials demonstrate this relationship?  Select all that apply.
a. 1 and 2                  b. 1 and 3                  c. 1 and 4                  d. 1 and 5
e. 2 and 4                  f. 2 and 5                   g. 3 and 4                  h. 3 and 5
 
 
 
 
 
 

How to Think About This Situation

In this question, you need to analyze a set of data to determine the effect that a quadrupling of Kelvin temperature has upon the volume of a gas (held at a constant pressure). The graphic below will provide some background to the topic. Study (and/or scan) the graphic and then return to the text that continues below the graphic.


 

Quadrupling Kelvin Temperature

When two quantities like volume and Kelvin temperature are directly proportional , a quadrupling of one quantity leads to a quadrupling of the other quantity.  So to determine the effect that this quadrupling has, you will have to look for two rows between which the temperature has been quadrupled. That is, one row's temperature must be four times another row's temperature. Inspect your data table and identify two such rows. Then you will notice that the volume of the row with the highest temperature is four times the volume of the row with the smallest temperature. 
 


Tired of Ads?
Go ad-free for 1 year