Vectors and Projectiles
Navigate to:
Review Session Home - Topic Listing
Vectors and Projectiles - Home ||
Printable Version ||
Questions and Links
Answers to Questions:
All ||
#1-9 ||
#10-45 ||
#46-55 ||
#56-72
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Part A: Multiple-Multiple Choice
1. Which of the following statements are true of scalars and vectors? List all that are TRUE.
- A vector quantity always has a direction associated with it.
- A scalar quantity can have a direction associated with it.
- Vectors can be added together; scalar quantities cannot.
- Vectors can be represented by an arrow on a scaled diagram; the length of the arrow represents the vector's magnitude and the direction it points represents the vector's direction.
Answer: AD
a. TRUE - Vectors are defined as quantities which are fully described by both their magnitude and direction. By definition, a vector has a direction associated with it. If it didn't, then it would NOT be a vector.
b. FALSE - Scalars are defined as quantities which are fully described by their magnitude alone. Scalars have no regard for direction and it is meaningless to associate a direction with such a quantity. If a quantity did have a direction associated with it, then that quantity would not be a vector.
c. FALSE - Both vectors and scalars can be added together. The rules for adding vectors together are unique to vectors and cannot be used when adding scalars together. The direction of a vector must be considered when adding two vectors together. Direction is of no importance when adding scalars.
d. TRUE - This is exactly the case and exactly what is done throughout the unit.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
2. Which of the following quantities are vectors? Include all that apply.
- distance traveled
- displacement
- average speed
- average velocity
- instantaneous velocity
- acceleration
Answer: BDEF
Of the five kinematic quantities listed here (distance, displacement, speed, velocity and acceleration), three of them are vectors. Displacement, velocity (both average and instantaneous), and acceleration all require the mention of a direction in order to fully describe the quantity.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
3. Numerical values and directions are stated for a variety of quantities. Which of these statements represent a vector description? Include all that apply.
- 20 meters, west
- 9.8 m/s/s
- 35 mi/hr, south
- 16 years old
- 60 minutes
- 3.5 m/s/s, south
- -3.5 m/s/s
- +20 degrees C
Answer: ACFG
Expressions of vector quantities would include a magnitude (number, value, etc.) and a direction. The direction could be described as being north, south, east, west or left, right, up, down. On occasion, a "+" or "-" is used to describe the direction. Since mathematical computations on calculators do not fare well with the typing of "south," a - sign is often substituted for a given direction. In the case of g, the units indicate an acceleration quantity. The "-" sign indicates a direction. One must be careful in assuming that a "+" or "-" sign is a sure sign of a quantity being a direction for other non-vector quantities can use such signs as well (as is the case in h).
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
4. Which of the following statements are true of vector addition, vector subtraction, and vector addition diagrams? List all that apply.
- Vectors A, B, and C are added together as A + B + C. If the order in which they are added is changed to C + B + A, then the result would be different.
- Vectors A, B, and C are added together as A + B + C. If the order in which they are added is reversed to C + B + A, then the result would be a vector with the same magnitude but the opposite direction.
- When constructing a vector diagram for A + B + C, it is not absolutely necessary that vectors B and C use the same scale that is used by vector A.
- The resultant in a vector addition diagram always extends from the head of the last vector to the tail of the first vector.
- If vectors A and B are added at right angles to each other, then one can be sure that the resultant will have a magnitude that is greater than the magnitudes of either one of the individual vectors A and B.
- If vectors A and B are added at right angles to each other, then one can be sure that the resultant will have a magnitude that is less than the arithmetic sum of the magnitudes of A and B.
- Vector addition diagrams cannot be used to determine the resultant when there is a vector subtraction operation.
Answer: EF
a. FALSE - Altering the order in which three vectors are added does not alter the result of the addition process. A + B + C = C + B + A. Each order of operation yields a resultant with the same magnitude and direction.
b. FALSE - As mentioned above in a, altering the order in which three vectors are added does not alter the result of the addition process. Reversing the order produces a resultant with the same magnitude and the same direction.
c. FALSE - When constructing a vector addition diagram, a scale must be chosen and adhered to. The scale which used to draw vector A must also be used for vectors B and C. One cannot switch horses in the middle of the stream.
d. FALSE - The resultant in a vector addition diagram is drawn from the tail of the first vector (the starting point) to the head of the last vector (the finishing point).
e. TRUE - Suppose that A = 3 units and B = 4 units and that the two vectors are directed at right angles to each other. The vector sum or resultant of A + B is 5 units, which is clearly greater than either one of the vectors being added. In general, the resultant in such a case will be represented on a vector addition diagram as the hypotenuse of a right triangle. The hypotenuse is always greater than the other two legs of the triangle. So this statement is always true.
f. TRUE - Suppose that A = 3 units and B = 4 units and that the two vectors are directed at right angles to each other. The vector sum or resultant of A + B is 5 units whereas the arithmetic sum is 7 units. In this case and in all cases, the vector sum of two right angle vectors will always be less than the arithmetic sum. That is, Sqrt(a2 + b2) will always be less than a + b.
g. FALSE - When a vector subtraction operation is performed, it is usually advisable to simply convert it into a vector addition operation. This is accomplished by adding the negative of the vector which is being subtracted. So A - B would be equivalent to A + (-B). By so doing, a vector addition diagram can be used to determine the resultant.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
5. Which of the following descriptions of moving objects accurately portray a projectile? List all that apply.
- an object which is moving through the air and not touching any surface
- a falling skydiver with an open parachute
- any object upon which air resistance is negligible
- a free-falling object
- an object upon which the only significant force is the force of gravity
- a falling feather
- a falling feather in a vacuum chamber
- a falling feather in a falling vacuum chamber.
Answer: DEGH
A projectile is an object upon which the only force is gravity. Air resistance must be negligible or nonexistent. Other forces resulting from people or things pulling or pushing, attached strings or contact with surfaces must not be present.
a. NO - A plane moves through the air and is not touching any surface. Yet, a plane is clearly not a projectile.
b. NO - A falling skydiver typically experiences considerable air resistance. It is popular to describe such skydivers as being in free fall. This is an erroneous use of the term.
c. NO - As you sit in your chair, air resistance is negligible. You are certainly not a projectile (at least, we hope not).
d. YES - A projectile is an object in free fall.
e. YES - An object upon which the only significant force is gravity fits the definition of a projectile (provided that significant means "having an influence").
f. NO - Falling feathers encounter air resistance which impedes the downward acceleration and causes the feather to fall at nearly a constant velocity.
g. YES - When a feather is allowed to fall in a vacuum, air resistance is eliminated and the feather can free fall.
h. YES - When a feather is allowed to fall in a vacuum and the vacuum is free-falling as well, air resistance is eliminated and an observer would notice that both the vacuum chamber and the feather are in free fall.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
6. Which of the following statements are true of projectiles? List all that apply.
- A projectile is a free-falling object.
- A projectile experiences negligible or no air resistance.
- A projectile must be moving in the downward direction.
- A projectile must be accelerating in the downward direction.
- A projectile does not have to have horizontal motion.
- A projectile could begin its projectile motion with a downward velocity.
- A projectile does not need to be "falling."
Answer: ABDEF and possibly G
a. TRUE - Free-falling objects, like projectiles, are objects upon which the only significant force is gravity.
b. TRUE - The only force on a projectile is gravity; air resistance must not be present or must not have an influence upon the motion of the projectile.
c. FALSE - Projectiles can be moving either upward or downward or at an angle to the vertical. They must however be accelerating downward, consistent with gravity's effect on an object.
d. TRUE - The force of gravity acts directly downwards upon an object, causing a downward acceleration. Any projectile must be accelerating downwards regardless of other features of its motion.
e. TRUE - A projectile could be moving strictly in a vertical direction with no horizontal motion. A ball thrown straight up in the air would be such a case.
f. TRUE - There is no rule about which direction a projectile must be moving at the instant it is projected. It could begin its motion with a initial downward velocity.
g. TRUE - The word "falling" can mean different things to different people. If "falling" involves moving in the downward direction at all instants in time, then a projectile does not need to be "falling." To many, "falling" means being pulled downward by gravity's force. In this case, a projectile must be "falling."
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
7. Which of the following statements are true of the horizontal motion of projectiles? List all that apply.
- A projectile does not have a horizontal velocity.
- A projectile with a rightward component of motion will have a rightward component of acceleration.
- The horizontal velocity of a projectile changes by 9.8 m/s each second.
- A projectile with a horizontal component of motion will have a constant horizontal velocity.
- The horizontal velocity of a projectile is 0 m/s at the peak of its trajectory.
- The horizontal velocity of a projectile is unaffected by the vertical velocity; these two components of motion are independent of each other.
- The horizontal displacement of a projectile is dependent upon the time of flight and the initial horizontal velocity.
- The final horizontal velocity of a projectile is always equal to the initial horizontal velocity.
- As a projectile rises towards the peak of its trajectory, the horizontal velocity will decrease; as it falls from the peak of its trajectory, its horizontal velocity will decrease.
- Consider a projectile launched from ground level at a fixed launch speed and a variable angle and landing at ground level. The horizontal displacement (i.e., the range) of the projectile will always increase as the angle of launch is increased from 0 degrees to 90 degrees.
- Consider a projectile launched from ground level at a fixed launch angle and a variable launch speed and landing at ground level. The horizontal displacement (i.e., the range) of the projectile will always increase as the launch speed is increased.
Answer: DFGHK
a. FALSE - Many projectiles are moving from left to right and from right to left as they simultaneously free fall. Such projectiles have a horizontal motion. While a projectile can have a horizontal motion, it cannot have a horizontal acceleration. Whatever motion which it has in the horizontal dimension, must be motion with a constant velocity.
b. FALSE - A projectile with a rightward motion (in addition to a vertical motion) will have a constant velocity in the rightward direction. This is to say that it has no horizontal acceleration.
c. FALSE - A projectile has a constant horizontal velocity. The vertical velocity will change by 9.8 m/s each second.
d. TRUE - Absolutely true! Projectiles are objects being acted upon by gravity alone. As such, there is a vertical acceleration but no horizontal acceleration. The horizontal velocity of a projectile is either zero or a constant nonzero value.
e. FALSE - The vertical velocity of a projectile is 0 m/s at the peak of its trajectory; but the horizontal component of the velocity at the peak is whatever the value was when first launched.
f. TRUE - For any two dimensional motion (whether projectile motion or riverboat problems or ...), perpendicular components of the motion are independent of each other. Any alteration in a vertical component will not effect the horizontal components of motion.
g. TRUE - The horizontal displacement (x) can be calculated with the formula x = vox • t, where vox is the initial horizontal velocity and t is the time. These are the two variables which effect the horizontal displacement of a projectile.
h. TRUE - Since there is no horizontal acceleration for a projectile, the initial horizontal velocity is equal to the final horizontal velocity.
i. FALSE - This is a true description for the vertical component of the velocity. The horizontal velocity is unchanging throughout the trajectory of a projectile.
j. FALSE - The range (or horizontal displacement) will increase as the angle is increased from 0 degrees to 45 degrees. The maximum range occurs at 45 degrees. As the angle is further increased to values greater than 45 degrees, the horizontal displacement decreases.
k. TRUE - As the launch speed is increased, the components of the initial velocity (both the horizontal and the vertical) increase as well. This causes the projectile to stay in the air for a longer period of time and to be moving faster in the horizontal direction. The result is that increased launch speeds always lead to increased horizontal displacements.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
8. Which of the following statements are true of the vertical motion of projectiles? List all that apply.
- The vertical component of a projectile's velocity is a constant value of 9.8 m/s.
- The vertical component of a projectile's velocity is constant.
- The vertical component of a projectile's velocity is changing.
- The vertical component of a projectile's velocity is changing at a constant rate.
- A projectile with an upward component of motion will have a upward component of acceleration.
- A projectile with an downward component of motion will have a downward component of acceleration.
- The magnitude of the vertical velocity of a projectile changes by 9.8 m/s each second.
- The vertical velocity of a projectile is 0 m/s at the peak of its trajectory.
- The vertical velocity of a projectile is unaffected by the horizontal velocity; these two components of motion are independent of each other.
- The final vertical velocity of a projectile is always equal to the initial vertical velocity.
- The vertical acceleration of a projectile is 0 m/s/s when it is at the peak of its trajectory.
- As a projectile rises towards the peak of its trajectory, the vertical acceleration will decrease; as it falls from the peak of its trajectory, its vertical acceleration will decrease.
- As a projectile rises towards the peak of its trajectory, the vertical acceleration is directed upward; as it falls from the peak of its trajectory, its vertical acceleration is directed downward.
- The peak height to which a projectile rises above the launch location is dependent upon the initial vertical velocity.
- As a projectile rises towards the peak of its trajectory, the vertical velocity will decrease; as it falls from the peak of its trajectory, its vertical velocity will decrease.
- Consider a projectile launched from ground level at a fixed launch speed and a variable angle and landing at ground level. The vertical displacement of the projectile during the first half of its trajectory (i.e., the peak height) will always increase as the angle of launch is increased from 0 degrees to 90 degrees.
- Consider a projectile launched from ground level at a fixed launch angle and a variable launch speed and landing at ground level. The vertical displacement of the projectile during the first half of its trajectory (i.e., the peak height) will always increase as the launch speed is increased.
Answer: CDFGHINPQ
a. FALSE - The vertical component of a projectile's velocity is constantly changing. It is the acceleration which has a value of 9.8 m/s/s.
b. FALSE - Projectiles are objects being acted upon by gravity alone. As such, there is a vertical acceleration; the vertical velocity is not constant, but changing.
c. TRUE - See part b above.
d. TRUE - A projectile has a vertical acceleration of 9.8 m/s/s throughout the entire trajectory. This acceleration value is constant. This means that the vertical velocity changes by the same amount - 9.8 m/s - during each second of its motion. There is a change in the vertical velocity by a constant amount.
e. FALSE - All projectiles experience a downward acceleration, whether they are moving upward or downward. The upward-moving projectiles have an upward velocity, but the actual velocity values are getting smaller; that is, the projectile is slowing down on the way to its peak.
f. TRUE - This is a true statement. It could also be said that a projectile with an upward component of motion also has a downward acceleration. All projectiles accelerate in the downward direction. Period.
g. TRUE - This is absolutely true .
h. TRUE - At the peak of its trajectory, a projectile is in the process of changing directions. The vertical velocity must change from a positive value (+ for upward) to a negative value (- for downward). This transition means that the value for the vertical velocity must at sometime be in between a + and - number. The in-between number is 0 m/s and this occurs at the peak.
i. TRUE - For any two dimensional motion (whether projectile motion or riverboat problems or ...), perpendicular components of the motion are independent of each other. Any alteration in a vertical component will not effect the horizontal components of motion.
j. FALSE - A projectile launched at an angle forms a parabolic trajectory. Suppose that one were to trace a projectile's motion forward in time from the peak and backwards in time from the peak. If done, one would find that the vertical velocity value has the same magnitude for equal amounts of times traced forward and backward from the peak. So for the same time before and after the peak, a projectile has the same speed. However, some projectiles are not launched from the same height at which they land. The final height is not the same as the initial height and as such the time to rise to the peak is not equal to the time to fall from the peak. In such instances, the initial vertical velocity is not equal to the final vertical velocity.
k. FALSE - No! No! No! The vertical velocity is 0 m/s at the peak and the vertical acceleration is -9.8 m/s/s throughout the entire trajectory.
l. FALSE - This would be a true description of the vertical velocity. But the vertical acceleration is a constant value of 9.8 m/s/s throughout the entire trajectory.
m. FALSE - Not only is the magnitude of the vertical acceleration a constant value throughout a projectile's trajectory, the direction is constant as well. Projectile's at all times regardless of any other variable will accelerate downwards at 9.8 m/s/s. This is perhaps the most important truth to digest about projectiles.
n. TRUE - The initial vertical velocity has an effect on the time taken by a projectile to rise towards its peak. It also effects the average speed of the projectile as it rises towards its peak. As a result, any alteration in the vertical velocity will alter the peak height of the projectile.
o. FALSE - Upward-rising projectiles have a downward acceleration; this means they are slowing down as they rise. The magnitude of their velocity is decreasing. Downward-moving projectiles also have a downward acceleration; this means they are speeding up. The magnitude of their velocity is increasing.
p. TRUE - An increase in the angle of launch (from 0 to 90 degrees) will always increase the vertical component of the initial velocity (viy). This increase in viy will lead to increased times for the projectile rising towards its peak. And an increased angle causes the projectile to move with a greater average speed during its path towards its peak. Both of these effects lead to the outcome that the peak height of a projectile will increase as the angle of launch increases from 0 to 90 degrees.
q. TRUE - As the launch speed is increased, the components of the initial velocity (both the horizontal and the vertical) increase as well. This causes the projectile to stay in the air for a longer period of time and to be moving faster in the vertical direction. The result is that increased launch speeds always lead to increased heights for projectiles.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
9. Which of the following statements are true of the time of flight for a projectile? List all that apply.
- The time that a projectile is in the air is dependent upon the horizontal component of the initial velocity.
- The time that a projectile is in the air is dependent upon the vertical component of the initial velocity.
- For a projectile which lands at the same height that it is projected from, the time to rise to the peak is equal to the time to fall from its peak to the original height.
- For the same upward launch angles, projectiles will stay in the air longer if the initial velocity is increased.
- Assume that a kicked ball in football is a projectile. If the ball takes 3 seconds to rise to the peak of its trajectory, then it will take 6 seconds to fall from the peak of its trajectory to the ground.
Answer: BCD
a. FALSE - The time for a projectile to rise vertically to its peak (and subsequently fall back to the ground) is dependent upon the initial vertical velocity. Alteration in the horizontal velocity will only cause the projectile to have a greater horizontal displacement (x).
b. TRUE - Absolutely true. Projectiles with a greater vertical component of initial velocity will be in the air for longer amount of times (assuming that the direction of viy is upward). An alteration in the viy value will alter the time of flight of the projectile, regardless of the direction of viy.
c. TRUE - For projectiles launched at upward angles and landing at the original height, the time to the rise to the peak equals the time to fall from the peak. If it takes 3 seconds to rise upward, it will take 3 seconds to fall.
d. TRUE - For a constant launch angle, an increase in the initial velocity (vi) will increase the vertical velocity (viy). This results in an increased time for the projectile to decelerate to 0 m/s as it rises towards its peak. So the projectile takes longer to get to the peak, longer to fall from the peak and overall is in the air for a longer time.
e. FALSE - Close, but very false. If it takes 3 seconds to rise to the peak, then it takes 3 seconds to fall from the peak; The 6 seconds is the total time of flight of the projectile.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Part B: Multiple Choice
10. If two displacement vectors of 6 meters and 8 meters (with varying directions) are added together, then the resultant could range anywhere between ___ meters and ___ meters.
|
a. 0, 48
|
b. 1.33, 48
|
c. 0, 14
|
d. 2, 14
|
|
e. ... nonsense! No such prediction can be made.
|
|
f. ... nonsense! A prediction can be made but none of these choices are correct.
|
Answer: D
The vector sum of 6.0 meters and 8.0 meters will be the greatest if they are added together in the same direction; that would produce a resultant of 14 meters. The vector sum of 6.0 meters and 8.0 meters will be the smallest if they are added together in the opposite direction; that would produce a resultant of 2.0 meters. An animation depicting the various resultants of 6.0 meters added to 8.0 meters at varying directions is shown on a separate page. See animation.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
11. Three vectors are added following the rules of vector addition. A fourth vector is drawn from the tail of the first vector to the head of the last vector. This fourth vector is referred to as ____.
|
a. the equilibrant
|
b. the hypotenuse
|
c. the resultant
|
d. a mistake
|
Answer: C
The resultant represents the result of adding two or more vectors. On a scaled vector addition diagram, the resultant is always drawn from the tail of the first vector to the head of the last vector.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
12. TRUE or FALSE:
The order in which vectors is added will effect the end result.
Answer: B
The order in which vectors is added does not effect the magnitude or direction of the resultant. A + B + C gives the same resultant as B + C + A and the same resultant as A + C + B. An animation depicting the the head-to-tail addition of five vectors in three different orders is shown on a separate page. See animation.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
13. Vector A is directed northward and vector B is directed eastward. Which of the following vector addition diagrams best represent the addition of vectors A and B and the subsequent resultant?
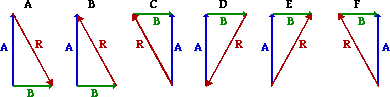
Answer: E
If the head-to-tail method (sometimes referred to as the triangle method) is being used, then the tail of B should be drawn starting at the head of A. Both diagrams D and E show this. Then the resultant should be drawn from the tail of A to the head of B (which is not shown in diagram D). There is also a parallelogram method for adding vectors. In this method, the tails of the two vectors are placed together (as in diagrams A and B). Then a parallelogram should be drawn with the two vectors forming the adjacent sides of the parallelogram. The resultant is drawn from the tails of the vectors to the opposite corner of the parallelogram. This is not done correctly on diagrams A and B. Diagrams C and F do not resemble any (accurate) vector addition method known to humankind.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
14. When adding vector B to vector A geometrically (or graphically) using the head to tail method, the resultant is drawn from ____ to the ____.
|
a. head of A, tail of B
|
b. tail of A, head of B
|
|
c. head of B, tail of A
|
d. tail of B, head of A
|
Answer: B
Adding vector B to vector A is equivalent to saying A + B. In such an instance, A is drawn first, then B is drawn with its tail at the head of A. Finally, the resultant is drawn from the tail of the first vector (A) to the head of the last vector (B).
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Use the following vector addition diagrams for Questions #15-#20.
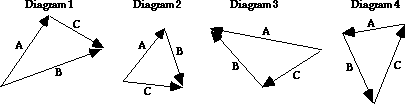
15. Which one of the following vector addition equations is shown in Diagram 1?
|
a. A + B = C
|
b. A + C = B
|
c. B + C = A
|
d. B + A = C
|
|
e. C + B = A
|
f. C + A = B
|
g. None of these
|
|
16. Which one of the following vector addition equations is shown in Diagram 2?
|
a. A + B = C
|
b. A + C = B
|
c. B + C = A
|
d. B + A = C
|
|
e. C + B = A
|
f. C + A = B
|
g. None of these
|
|
17. Which one of the following vector addition equations is shown in Diagram 3?
|
a. A + B = C
|
b. A + C = B
|
c. B + C = A
|
d. B + A = C
|
|
e. C + B = A
|
f. C + A = B
|
g. None of these
|
|
18. Which one of the following vector addition equations is shown in Diagram 4?
|
a. A + B = C
|
b. A + C = B
|
c. B + C = A
|
d. B + A = C
|
|
e. C + B = A
|
f. C + A = B
|
g. None of these
|
|
Answers to questions #15-#18:
15. B
16. A
17. E
18. G
Vectors are added by a head-to-tail method and the resultant is drawn from the tail of the first vector to the head of the last vector. So if two vectors are added - say B is added to A (as in A + B) - then first A is drawn and the tail of B is placed at the head of A. The resultant is drawn with its tail at the tail of A and its head at the head of B. Thus, when the tails of two vectors are seen connected, one of the vectors is the resultant and the other is the first vector being added. And when the heads of two vectors are seen connected, one of the vectors is the resultant and the other is the second vector being added. These principles can be applied to answer the above questions.
Note that in question 18, there is not a single vector which is drawn from the tail of one vector to the head of another vector. Thus, no resultant is drawn. One could say that the diagram shows that A + B + C = 0.
19. Consider the magnitude and direction of vectors A and B as shown in Diagram 1 above. Which one of the following diagrams would represent B - A = R?
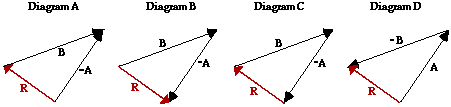
Answer: B
The subtraction of A from B is equivalent to adding the negative of A to B. That is, B - A = B + (-A). The negative of a vector is simply the same vector drawn in the opposite direction. The correct answer should be a diagram which shows B being drawn first. Then at the head of B, the tail of a vector pointing in the opposite direction of A should be drawn. This is shown in both diagrams B and C. The resultant should then be drawn from the tail of B to the head of -A. This is not shown in diagram C.
20. Consider the magnitude and direction of vectors B and C as shown in Diagram 2 above. Which one of the following diagrams would represent C - B = R?
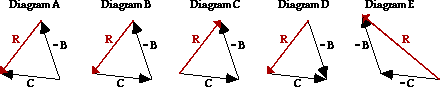
Answer: C
The subtraction of B from C is equivalent to adding the negative of B to C. That is, C - B = C + (-B). The negative of a vector is simply the same vector drawn in the opposite direction. The correct answer should be a diagram which shows C being drawn first. Then at the head of C, the tail of a vector pointing in the opposite direction of B should be drawn. This is shown in both diagrams B and C. The resultant should then be drawn from the tail of C to the head of -B. This is not shown in diagram B.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
21. The vector sum (magnitude only) of 25.0 m, north + 18.0 m, East is ___ m.
|
a. 7.00
|
b. 21.5
|
c. 30.8
|
d. 35.8
|
|
e. 43.0
|
f. 54.2
|
g. 949
|
h. None of these
|
Answer: C
These two vectors are directed at right angles to each other. In such instances, the vector sum can be determined using the Pythagorean theorem. The resultant (R) is equal to the square root of the sum of the squares of the two vectors being added. That is, R = Sqrt (A2 + B2) where A and B are the two vectors being added together. Thus,
R = Sqrt [ (25.0 m)2 + (18.0 m)2 ] = Sqrt [ (625 m2) + (324 m2) ] = Sqrt (949 m2)
R = 30.805 m = ~30.8 m
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
22. The vector sum (magnitude only) of 32.0 m, north + 41.0 m, west is ___ m.
|
a. 9.00
|
b. 36.5
|
c. 38.0
|
d. 52.0
|
|
e. 73.0
|
f. 128
|
g. 2.70 x 103
|
h. None of these
|
Answer: D
Like question #21 above, these two vectors are directed at right angles to each other. The Pythagorean theorem can be used to determine the resultant of their sum. The resultant (R) is equal to the square root of the sum of the squares of the two vectors being added.
R = Sqrt (A2 + B2)
R = Sqrt [ (32.0 m)2 + (41.0 m)2 ] = Sqrt [ (1024 m2) + (1681 m2) ] = Sqrt (2705 m2)
R = 52.010 m = ~52.0 m
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Use the diagram below to answer Questions #23-#28. Each square on the diagram represents a 20-meter x 20-meter area.
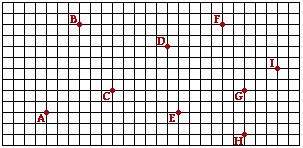
23. If a person walks from D to H to G to C, then the distance walked is ____ meters.
|
a. 128
|
b. 180
|
c. 401
|
d. 460
|
|
e. 480
|
f. 533
|
g. 620
|
h. None of these
|
Answer: F
Distance is a scalar quantity and adding together three scalars is as simple as adding the three numbers arithmetically. However, since the first of the three legs of this trip is not due East, West, North or South, determining the distance for this leg requires that the Pythagorean theorem is used. That is, walking from D to H is equivalent to walking 140 meters east (7 squares) and 160 meters (8 squares) south. The distance from D to H is thus 212.6 meters - Sqrt [ (140 m)2 + (160 m)2 ]. The walk from H to G is a distance of 80 meters (4 squares) and the walk from G to C is 240 meters (12 squares). The total distance walked is the sum of 212.6 m + 80 m + 240 m = 532.6 m = ~533 m.
24. If a person walks from D to H to G to C, then the magnitude of the displacement is ____ meters.
|
a. 128
|
b. 180
|
c. 401
|
d. 460
|
|
e. 480
|
f. 533
|
g. 620
|
h. None of these
|
Answer: A
Displacement is a vector quantity which indicates how far our of place an object is at the end of the motion relative to the beginning of the motion. Displacement does not depend upon the path taken from the beginning position to the final position, but only upon the distance of a vector drawn from start to finish. The resultant for the three segments of this walk is simply drawn from D to C. The distance of this resultant is found by using the Pythagorean theorem. The resultant stretches south 80 meters (4 squares) and west 100 meters (5 squares). The resultant has a magnitude of Sqrt [ (80 m)2 + (100 m)2 ] or 128 meters.
25. If a person walks from D to H to G to C, then the direction of the displacement is ___ degrees (as measured counterclockwise from East).
|
a. 39
|
b. 51
|
c. 53
|
d. 217
|
|
e. 219
|
f. 231
|
g. 233
|
h. None of these
|
Answer: E
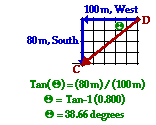 As discussed in the previous problem, the resultant stretches south 80 meters (4 squares) and west 100 meters (5 squares). This is shown in the diagram at the right. These two parts of the resultant vector can be combined with a trigonometric function to determine the angle theta. The angle theta is approximately 38.7 degrees. This is not the direction of the resultant displacement but simply the angle between the displacement vector and due West. The convention used to express the direction of the vector is to measure the counterclockwise angle of rotation from due East. So in this instance, the direction is 180 degrees + 38.7 degrees or ~219 degrees.
As discussed in the previous problem, the resultant stretches south 80 meters (4 squares) and west 100 meters (5 squares). This is shown in the diagram at the right. These two parts of the resultant vector can be combined with a trigonometric function to determine the angle theta. The angle theta is approximately 38.7 degrees. This is not the direction of the resultant displacement but simply the angle between the displacement vector and due West. The convention used to express the direction of the vector is to measure the counterclockwise angle of rotation from due East. So in this instance, the direction is 180 degrees + 38.7 degrees or ~219 degrees.
26. If a person walks from H to E to C to G, then the distance walked is ____ meters.
|
a. 80.
|
b. 240.
|
c. 253
|
d. 333
|
|
e. 493
|
f. 560.
|
g. 640.
|
h. None of these
|
Answer: E
Distance is a scalar quantity and adding together three scalars is as simple as adding the individual distances of the three legs is as simple as adding the distances. arithmetically. The problem is simplified in that the first two legs of the trip are along the same line segment. The stop at E can be disregarded since its is merely a point on the line from location H to location C. That is HE + EC = HC. Since the HC line segment does not stretch not due East, West, North or South, determining the distance for this leg requires that the Pythagorean theorem is used. That is, walking from H to C is equivalent to walking 240 meters west (12 squares) and 80 meters (4 squares) north. The distance from D to H is thus 253.0 meters - Sqrt [ (240 m)2 + (80 m)2 ]. The walk from H to E to C is a distance of 253 meters and the walk from C to G is 240 meters (12 squares). The total distance walked is the sum of 253 m + 240 m = ~493 m.
27. If a person walks from H to E to C to G, then the magnitude of the displacement is ____ meters.
|
a. 80.
|
b. 240.
|
c. 253
|
d. 333
|
|
e. 493
|
f. 560.
|
g. 640.
|
h. None of these
|
Answer: A
Displacement is a vector quantity which indicates how far our of place an object is at the end of the motion relative to the beginning of the motion. Displacement does not depend upon the path taken from the beginning position to the final position, but only upon the distance of a vector drawn from start to finish. The resultant for the three segments of this walk is simply drawn from H to G. The distance of this resultant is simply 80 meters (4 squares), north.
28. If a person walks from H to E to C to G, then the direction of the displacement is ___ degrees (as measured counterclockwise from East).
|
a. 0
|
b. 18.
|
c. 72
|
d. 90.
|
|
e. 108
|
f. 162
|
g. 342
|
h. None of these
|
Answer: D
The final destination G is due North of the initial location. Thus, the displacement is directed north (at 90 degrees).
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
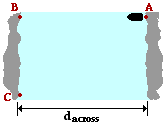
Use the following diagram for Questions #29-#33. In the diagram, a riverboat is shown starting at position A on the east bank of a river. The boat heads towards position B (a point directly across the river from A) with a speed of 3.8 m/s. But because of a current with a speed of 1.8 m/s, the boat lands on the west bank of the river at position C, a location downstream from B. The width of the river (dacross) is 86.4 meters.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
29. The magnitude of the resultant velocity of the boat is ____ m/s.
|
a. 1.8
|
b. 2.0
|
c. 3.4
|
d. 3.8
|
|
e. 4.2
|
f. 5.6
|
g.11
|
h. None of these
|
Answer: E
This is a case of a boat crossing a river by use of its motor. The motor allows the boat to travel 3.8 meters towards the opposite shore in every second. The river is flowing south, carrying the boat a distance of 1.8 meters down the river every second. The resultant velocity is simply the vector sum of these two individual velocities. Since these two velocities are at right angles to each other, the vector sum can be determined using the Pythagorean theorem.
vresultant2 = (3.8 m/s)2 + (1.8 m/s)2
vresultant = Sqrt[ (3.8 m/s)2 + (1.8 m/s)2 ]
vresultant = 4.2 m/s
30. The direction of the resultant velocity of the boat is ____ m/s.
|
a. 0
|
b. 18.
|
c. 712
|
d. 90.
|
|
e. 108
|
f. 162
|
g. 342
|
h. None of these
|
Answer: H
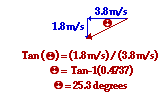 The direction of the resultant velocity is in a southeastern direction. That puts the vector in the third quadrant with a direction somewhere between 180 degrees and 270 degrees. The exact angle can be determined if the angle theta is found using trigonometry. Theta is the angle that the resultant velocity (the red vector in the diagram at the right) makes with due West. This angle can be found suing the tangent function. The work is shown at the right.
The direction of the resultant velocity is in a southeastern direction. That puts the vector in the third quadrant with a direction somewhere between 180 degrees and 270 degrees. The exact angle can be determined if the angle theta is found using trigonometry. Theta is the angle that the resultant velocity (the red vector in the diagram at the right) makes with due West. This angle can be found suing the tangent function. The work is shown at the right.
The angle theta is found to be 25.3 degrees. The actual direction as measured as the counterclockwise angle of rotation with due East is 180 degrees plus 25.3 degrees. This would be 205.3 degrees.
31. The time required for the boat to cross the 86.4 m wide river is ___ seconds.
|
a. 4.2
|
b. 15
|
c. 21
|
d. 23
|
|
e. 48
|
f. None of these
|
|
Answer: D
The motor allows the boat to travel 3.8 meters towards the opposite shore in every second. The boat has 86.4 meters to travel from shore to shore. (The presence of the current does not alter the width or shore-to-shore distance.) The time to cross the river can be calculated from the river width and the boat velocity using the v = d/t equation. Rearranging the equation to solve for t yields
t = d/v
t = (86.4 m) / (3.8 m/s) = ~23 s (unrounded 22.7 s)
32. Location C is the location where the boat ultimately lands on the opposite shore. What is the distance from location B to location C.
|
a. 37
|
b. 41
|
c. 78
|
d. 86
|
|
e. 96
|
f. 180
|
g. 2.0 x 102
|
h. None of these
|
Answer: B
The distance that location C is downstream from B is mathematically related to the speed of the river and the time to cross the river. The distance can be calculated by multiplying the current speed by the time to cross the river.
ddownstream = vriver • t
ddownstream = (1.8 m/s) • (22.7 s)
ddownstream = ~41 m
33. If the current on a particular day was flowing with two times the velocity, then the time to cross the river would be ____.
|
a. two times greater
|
b. one-half as great
|
|
c. greater, but not two times greater
|
d. less, but not one-half the original time
|
|
e. the same as it was when the current flowed at 1.8 m/s.
|
Answer: E
For this situation of a boat heading straight across the river, the current velocity is directed perpendicular to the boat velocity. These two components of the boats resulting motion are independent of each other. The boat velocity makes the sole contribution to the ability of the boat to cross the river. The river velocity only carries the boat southward down the river. So an alteration of the river velocity would have no effect on the time required for the boat to cross the river.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
34. An object is undergoing free fall motion. As it falls, the object's ____.
|
a. speed increases
|
b. acceleration increases
|
|
c. both of these
|
d. none of these
|
Answer: A
As an object free-falls, its velocity (and also its speed) changes by approximately 10 m/s every second. This means that the acceleration is a constant value of 10 m/s/s. An object has a changing speed (or velocity) and a constant acceleration if the speed changes by the same amount (a "constant amount") in each consecutive second of its motion.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
35. A football is kicked into the air at an angle of 45 degrees with the horizontal. At the very top of the ball's path, its velocity is _______.
|
a. entirely vertical
|
b. entirely horizontal
|
|
c. both vertical and horizontal
|
d. not enough information given to know.
|
Answer: B
As a projectile rises towards its peak, its horizontal velocity remains constant while its vertical velocity decreases. At its peak, its vertical velocity becomes 0 m/s. At this instant in time, the velocity is entirely horizontal; there is no vertical component to the velocity.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
36. A football is kicked into the air at an angle of 45 degrees with the horizontal. At the very top of the ball's path, its acceleration is _______. (Neglect the effects of air resistance.)
|
a. entirely vertical
|
b. entirely horizontal
|
|
c. both vertical and horizontal
|
d. not enough information given to know.
|
Answer: A
As a projectile rises towards its peak, its horizontal velocity remains constant while its vertical velocity decreases. This is to say that the acceleration of the object is vertical, not horizontal. At the peak and everywhere throughout the trajectory, there is a vertical (downward) acceleration. In fact, a projectile is an object upon which the only force is gravity. This force causes an acceleration which is in the same direction as the force - downward.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
37. A football is kicked into the air at an angle of 45 degrees with the horizontal. At the very top of the ball's path, the net force acting upon it is _______. (Neglect the effects of air resistance.)
|
a. entirely vertical
|
b. entirely horizontal
|
|
c. both vertical and horizontal
|
d. not enough information given to know.
|
Answer: A
A projectile is an object upon which the only force is gravity. Since no other forces act upon the object, the net force would be downward.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
38. At what point in its path is the horizontal component of the velocity (vx) of a projectile the smallest?
|
a. The instant it is thrown.
|
b. Halfway to the top.
|
c. At the top.
|
|
d. As it nears the top.
|
e. It is the same throughout the path.
|
Answer: E
As a projectile rises towards its peak, its horizontal velocity remains constant while its vertical velocity decreases. This is to say that the acceleration of the object is vertical, not horizontal. Having a constant horizontal velocity, there is no point along the trajectory where the vx value is smaller than at other points.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
39. At what point in its path is the vertical component of the velocity (vy) of a projectile the smallest?
|
a. The instant it is thrown.
|
b. Halfway to the top.
|
c. At the top.
|
|
d. As it nears the top.
|
e. It is the same throughout the path.
|
Answer: C
As a projectile rises towards its peak, its horizontal velocity remains constant while its vertical velocity decreases. During the upward portion of its trajectory, the vy continuously decreases until it becomes 0 m/s at the peak. Thus, the vy is as small as it will ever be when it is at the peak of the trajectory.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
40. An airplane that flies at 100 km/h in a 100 km/h hurricane crosswind has a speed (relative to the ground) of ____.
|
a. 0 km/h
|
b. 100 km/h
|
c. 141 km/h
|
d. 200 km/h
|
Answer: C
When an object such as a plane or a boat moves within a medium which is moving relative to the ground, the speed of the plane or boat (as measured by its speedometer) will not be the same as the speed as measured by a person on the ground (ground speed). The ground speed (i.e., resultant velocity produced by the combination of the plane's speed and the wind speed) can be determined by adding the plane speed and wind speed as vectors. In this case, the two vectors are at right angles, so the resultant velocity can be determined using the Pythagorean theorem.
R2 = (100 km/hr)2 + (100 km/hr)2
R = SQRT( (100 km/hr)2 + (100 km/hr)2 )
R = 141 km/hr
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
41. An airplane moves at 141 km/h toward the northeast (45 degrees). What is its component velocity in the northward direction?
|
a. 41 km/h
|
b. 100 km/h
|
c. 110 km/h
|
d. 141 km/h
|
Answer: B
To determine the component of a vector in a given direction, vector resolution principles must be applied. This velocity vector is directed at 45 degrees and has magnitude of 141 km/hr. The vertical component (northward) of this vector can be found using the sine function.
vy = v * sine(theta)
vy = (141 km/hr)*sine(45 degrees)
vy = 100 km/hr
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
42. Roll a bowling ball off the edge of a table. As it falls, its horizontal component of velocity ___.
|
a. decreases
|
b. remains constant
|
c. increases
|
Answer: B
Once the ball leaves the table's edge, it becomes a projectile. As it falls, its horizontal velocity remains constant while its vertical velocity decreases. This is to say that the acceleration of the object is vertical, not horizontal. In fact, a projectile is an object upon which the only force is gravity. This force causes an acceleration which is in the same direction as the force - downward.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
43. A bullet is fired horizontally and hits the ground in 0.5 seconds. If it had been fired with twice the speed in the same direction, it would have hit the ground in ____. (Assume no air resistance.)
|
a. less than 0.5 s.
|
b. more than 0.5 s.
|
c. 0.5 s.
|
Answer: C
Once the bullet leaves the muzzle, it becomes a projectile (assuming no air resistance). As it falls, its horizontal velocity remains constant while its vertical velocity decreases. The force of gravity acts upon the bullet to cause its downward acceleration. The motion of the bullet in the downward direction is independent of the motion in the horizontal direction. That is to say, any alteration in a horizontal aspect of its motion will not effect the motion in the vertical direction. The time to fall vertically to the ground is not effected by the horizontal speed of the projectile. It would still take 0.5 seconds to fall to the ground from this height regardless of the horizontal speed.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
44. A projectile is launched at an angle of 15 degrees above the horizontal and lands down range. For the same speed, what other projection angle would produce the same downrange distance?
|
a. 30 degrees.
|
b. 45 degrees.
|
c. 50 degrees.
|
d. 75 degrees
|
e. 90 degrees.
|
Answer: D
For projectiles launched at angles, a launch angle of 45 degrees will provide the largest horizontal displacement. Any two launch angles which are separated from 45 degrees by the same amount (for example, 40 degrees and 50 degrees, 30 degrees and 60 degrees and 15 degrees and 75 degrees) will provide the same horizontal displacement.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
45. Two projectiles are fired at equal speeds but different angles. One is fired at angle of 30 degrees and the other at 60 degrees. The projectile to hit the ground first will be the one fired at (neglect air resistance) ____.
|
a. 30 degrees
|
b. 60 degrees
|
c. both hit at the same time
|
Answer: A
For projectiles launched at angles, a launch angle of 45 degrees will provide the largest horizontal displacement. Launch angles greater than 45 degrees result in large vertical components of velocity; these stay in the air longer and rise to higher heights. Launch angles less than 45 degrees result in small vertical components of velocity; these do not rise as high and end up falling to the ground in shorter times.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Part C: Diagramming and Analysis
46. Express the direction of each of the following vectors in the diagram below.
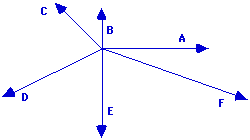
|
A: 0 deg
|
B: 90 deg
|
C: 135 deg
|
D: 205 deg
|
E: 270 deg
|
F: 340 deg
|
Answer: See answers above.
The convention that the direction of a vector is measured as the counterclockwise angle of rotation of that vector from due east is used. As such, an eastward vector (A) has a direction of 0 degrees; a northward vector (B) has a direction of 90 degrees; and a southward vector (E) has a direction of 270 degrees. A protractor must be used to determine the directions of C, D and F. Vector C is in the second quadrant, so its direction is between 90 degrees and 180 degrees. The angle between East and vector C can be measured. Vector D is in the third quadrant; its direction is between 180 degrees and 270 degrees. A protractor can be used to measure the angle between West (180 degrees) and vector D. This angle measure can be added to 180 degrees to get the answer. A similar procedure is repeated with Vector F.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
47. In the following diagrams, two vectors are being added and the resultant is drawn. For each diagram, identify which vector is the resultant and write the equation (e.g., A + B = C).
|
a.
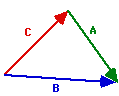
The resultant is vector B.
The equation is C + A = B.
|
b.

The resultant is vector B.
The equation is A + C = B.
|
|
c.
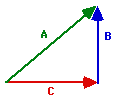
The resultant is vector A.
The equation is C + B = A.
|
d.

The resultant is vector C.
The equation is A + B = C.
|
Answer: See answers above.
Vectors are added by a head-to-tail method and the resultant is drawn from the tail of the first vector to the head of the last vector. So if two vectors are added - say B is added to A (as in A + B) - then first A is drawn and the tail of B is placed at the head of A. The resultant is drawn with its tail at the tail of A and its head at the head of B. Thus, when the tails of two vectors are seen connected, one of the vectors is the resultant and the other is the first vector being added. And when the heads of two vectors are seen connected, one of the vectors is the resultant and the other is the second vector being added. These principles can be applied to answer the above questions.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
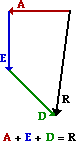
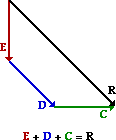
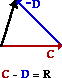
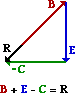
48. Consider the five vectors shown below; they are labeled A, B, C, D and E.
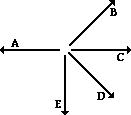
Use the stated magnitude and direction of these vectors to sketch a vector addition diagram for the following vector operations and determine the resultant. You only need to construct an approximate sketch; there is no need to do a scaled diagram.
|
A + B + C

|
A + C + B
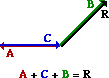
|
A + E + D
|
|
E + D + C
|
C - D
|
B + E - C
|
Answer: See diagrams above.
Vectors are added using a head-to-tail method. The magnitude and direction of the vectors are maintained when the addition (and subtraction) operations are performed. In your sketched diagrams, you should be sure to:
- place the tail of the second vector in the equation at the head of the first vector in the equation
- label each vector (A, B, C ...)
- draw the resultant from the tail of the first vector to the head of the last vector
- label the resultant
A subtraction operation is the same as adding the negative of a vector. So if D is to be subtracted from C, then simply add a vector whose magnitude is the same as D but is directed in the opposite direction.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
49. A hiker's motion can be described by the following three displacement vectors.
22.0 km, 45 degrees + 16.0 km, 135 degrees + 12.0 km, 270 degrees
Add the three displacement vectors using the head-to-tail method of vector addition. Then answer the following two questions.
(a) What is the distance walked by the hiker?
(b) What is the resulting displacement of the hiker?
Answer: Distance = 50.0 km; Displacement = 15.5 km, 74 degrees
Distance is a scalar quantity and does not regard the direction of the individual legs of the hike as being important. So the distance is simply the arithmetic sum of the distances of the three individual legs of the hike. Distance = 22.0 km + 16.0 km + 12.0 km = 50.0 km
Displacement is a vector quantity which is equal to the vector sum of the individual displacements of the three-legged hike. A scaled vector addition diagram serves as a useful tool in determining this sum. A convenient scale (such as 1 cm = 4 km or 1 cm = 5 km or 1 cm = 8 km) is chosen and the vectors are added using the head-to-tail method. This is shown in the diagram below.

Once the resultant is drawn, the magnitude (in cm) can be measured and the scale can be used to convert to kilometers. The direction can be measured from the diagram as the counterclockwise angle of rotation from due East. The displacement is 15.5 km at 74 degrees.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
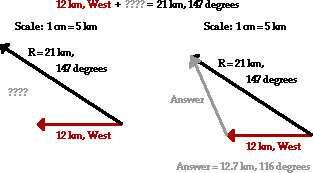
50. Solve the following problem using a vector addition diagram.
A hiker hikes for 12.0 km, west. She then makes a turn and hikes in a straight line until she reaches her final destination. If the resultant displacement is 21.0 km at 147 degrees, then what was the displacement (magnitude and direction) of the second leg of the hike?
Answer: 12.7 km, 116 degrees
This is a unique problem in that the resultant is given and one of the two vectors is given. The problem requests the magnitude and direction of the second vector. In the diagram below, the first vector is drawn and the resultant is drawn beginning at the tail of the first vector. The second vector would stretch from the head of the first vector to the head of the resultant. This can be drawn in the diagram and its magnitude and direction can be determined.
 [ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
51. Consider the trajectory diagram shown below for a horizontally launched projectile. On the diagram, draw vector arrows representing the vx and vy velocity components during the course of the motion. The length of the arrows should represent the magnitude of the velocity components. Label each component. (Note that the velocity components are already shown for the first position.)
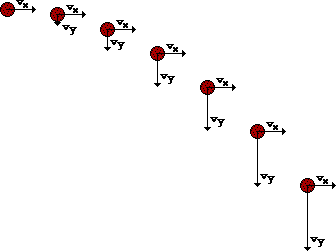
Answer: See diagram above.
A projectile has a downward acceleration. There is no horizontal acceleration; rather, the horizontal velocity remains constant. Thus, the vx vector is of constant length (i.e., magnitude) throughout the trajectory. The downward acceleration means that the vy vector will be changing. As the object falls, it speeds up. Thus, the vy vector increases its length (i.e., magnitude) throughout the trajectory. This is shown in the diagram above.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
52. Consider the trajectory diagram shown below for a non-horizontally launched projectile. On the diagram, draw vector arrows representing the vx and vy velocity components during the course of the motion. The length of the arrows should represent the magnitude of the velocity components. Label each component. (Note that the velocity components are already shown for the first position.)
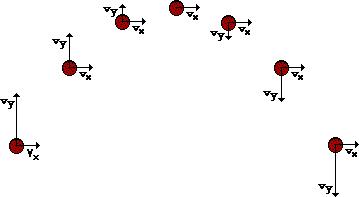
Answer: See diagram above.
A projectile has a constant horizontal velocity and a changing vertical velocity. The changing vertical component of velocity is consistent with the fact that there is a vertical acceleration. As the projectile rises towards the peak of its trajectory, the vertical velocity decreases until it reaches 0 m/s at the very peak of the trajectory. As the projectile rises towards the peak of its trajectory, the vertical velocity increases. The vertical velocity upon falling is of the same magnitude and the opposite direction as any corresponding location of the same height during the rising motion. The horizontal velocity remains constant throughout the trajectory. These principles are shown in the diagram above.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
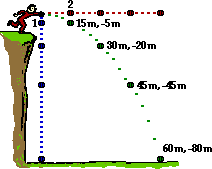
53. Consider the diagram below. The blue path represents the trajectory of a projectile dropped from rest from the top of the path. (Each ball location represents the location after a 1-second interval of time.) The red path represents the trajectory of the same ball thrown horizontally in the absence of gravity. Construct a third path accurately showing the trajectory of a projectile launched with the same horizontal speed as the red ball. Show the location during the first four seconds of motion. Finally, the (x, y) coordinate position of location 1 is (0 m, -5 m) and the (x, y) coordinate position of location 2 is (15 m, 0 m). Determine the (x, y) coordinate positions of the four locations in your trajectory. (Assume g ~10 m/s/s.)
Answer: See diagram above.
A projectile such as this has both a horizontal and vertical component of motion. These two components of motion are independent of each other. The strictly vertical motion of the ball (the blue path above) depicts the vertical displacement at 1-second intervals. If a horizontal motion is imparted to the ball, its vertical displacement will not be effected since these two components are independent of each other. The strictly horizontal motion of the ball (the red path above) portrays the motion of the ball in the absence of gravity. The presence of gravity would cause the ball to accelerate downward. This vertical motion does not effect the horizontal motion since these two components are independent of each other. The green path represents the motion of the projectile when gravity acts upon it and a horizontal motion is imparted to it. The horizontal location at each 1-second instant in time is the same position as it was in the red path above it. And the vertical location at each 1-second instant in time is the same position as it was in the blue path to the left of it.
The actual coordinate positions can be determined using the kinematic equations and the given time. For the x-coordinate, the displacement is the same each second - that is, there is a constant horizontal velocity. Since location 2 has a x-coordinate of 15 m, each consecutive location will be 15 m further than the one before it. The y-coordinate is determined by using the 0.5•a•t2 expression with times of 1 s, 2 s, 3 s, and 4 s. The simplification that g is ~10 m/s2 is used to simplify the math and highlight the concept.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
54. Consider the map of the United States at the right. Given the scale that 1 cm = 500 km, use a protractor and a ruler to determine the displacement of a traveler for the listed trips. A couple trips include two "legs." Indicate both the magnitude and the direction (using the counterclockwise from East convention) for the resultant displacement vector of the traveler.
|
Trip
|
Magnitude
|
Direction
|
|
a. From Chicago (CHI) to Washington, DC (WSH)
|
|
|
|
b. From Chicago (CHI) to Seattle (SEA)
|
|
|
|
c. From Jackson Hole (JXH) to Houston (HST)
|
|
|
|
d. From Denver (DEN) to Phoenix (PHX) to Washington (WSH)
|
|
|
|
e. From Salt Lake City (SLC) to Reno (REN) to Miami (MIA)
|
|
|
Answer: See table above.
a. The distance from CHI to WSH is ~2.6 cm. According to scale, this would be equivalent to ~1300 km. The direction of this displacement vector is measured as the counterclockwise angle of rotation from due East - 341 degrees.
b. The distance from CHI to SEA is ~6.7 cm. According to scale, this would be equivalent to ~3400 km. The direction of this displacement vector is measured as the counterclockwise angle of rotation from due East - 165 degrees.
c. The distance from JHX to HST is ~4.3 cm. According to scale, this would be equivalent to ~220 km. The direction of this displacement vector is measured as the counterclockwise angle of rotation from due East - 312 degrees.
d. This is a two-legged trip. Yet the displacement only depends upon the initial and final locations. When the traveler is done with the trip, he/she is displaced by an amount equal to the distance from DEN to WSH. This is ~6.4 cm on the map. According to scale, this would be equivalent to ~3200 km. The direction of this displacement vector is measured as the counterclockwise angle of rotation from due East - 356 degrees.
e. Again, the displacement only depends upon the initial and final locations. When the traveler is done with the trip, he/she is displaced by an amount equal to the distance from SLC to MIA. This is ~7.7 cm on the map. According to scale, this would be equivalent to ~3900 km. The direction of this displacement vector is measured as the counterclockwise angle of rotation from due East - 334 degrees.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
55. A cannonball is launched from the top of a 125-meter high cliff with an initial horizontal speed of 20 m/s. The (x, y) coordinate position of the launch location is designated as the (0, 0) position. Determine the (x, y) coordinate positions of the cannonball at 1-second intervals during its path to the ground. Assume g = ~10 m/s/s, down.
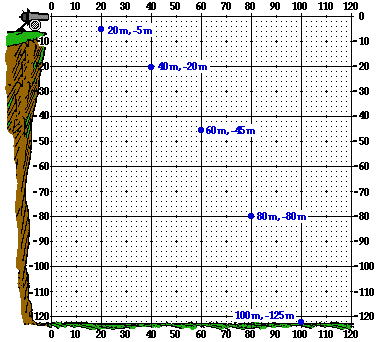
Answer: See diagram above.
The horizontal speed is 20 m/s throughout the trajectory. So the projectile will move a distance of 20 meters in each second. This horizontal displacement is combined with a falling motion which drops the projectile some vertical distance below the initial height of the cannon. The vertical displacement is given by the 0.5•a•t2 expression where the a value is stated as ~10 m/s2. Times of 1 s, 2 s, 3 s, etc. can be substituted into this expression to determine the vertical displacements of -5 m, -20 m, -45 m, -80 m, -125 m.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Part D: Problem-Solving
56. In the Vector Addition Lab, Anna starts at the classroom door and walks:
- 2.0 meters, West
- 12.0 meters, North,
- 31.0 meters, West,
- 8.0 meters, South
- 3.0 meters, East
Using either a scaled diagram or a calculator, determine the magnitude and direction of Anna's resulting displacement.
Answer: 30.3 meters, 172 degrees
To insure the most accurate solution, this problem is best solved using a calculator and trigonometric principles. The first step is to determine the sum of all the horizontal (east-west) displacements and the sum of all the vertical (north-south) displacements.
Horizontal: 2.0 meters, West + 31.0 meters, West + 3.0 meters, East = 30.0 meters, West
Vertical: 12.0 meters, North + 8.0 meters, South = 4.0 meters, North
The series of five displacements is equivalent to two displacements of 30 meters, West and 4 meters, North. The resultant of these two displacements can be found using the Pythagorean theorem (for the magnitude) and the tangent function (for the direction). A non-scaled sketch is useful for visualizing the situation.
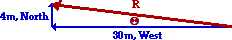
Applying the Pythagorean theorem leads to the magnitude of the resultant (R).
R2 = (30.0 m)2 + (4.0 m)2 = 916 m2
R = Sqrt(916 m2)
R = 30.3 meters
The angle theta in the diagram above can be found using the tangent function.
tangent(theta) = opposite/adjacent = (4.0 m) / (30.0 m)
tangent(theta) = 0.1333
theta = invtan(0.1333)
theta = 7.59 degrees
This angle theta is the angle between west and the resultant. Directions of vectors are expressed as the counterclockwise angle of rotation relative to east. So the direction is 7.59 degrees short of 180 degrees. That is, the direction is ~172 degrees.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
57. In a grocery store, a shopper walks 36.7 feet down an aisle. She then turns left and walks 17.0 feet straight ahead. Finally, she turns right and walks 8.2 feet to a final destination. (a) Determine the magnitude of the overall displacement. (b) Determine the direction of the displacement vector relative to the original line of motion.
Answer: (a) 48.0 feet; (b) 21 degrees from the original line of motion
This problem is best approached using a diagram of the physical situation. The three displacements are shown in the diagram below on the left. Since the three displacements could be done in any order without effecting the resulting displacement, these three legs of the trip are conveniently rearranged in the diagram below on the right.
Now it is obvious from the diagram on the right that the three displacement vectors are equivalent to two perpendicular displacement vectors of 44.9 feet and 17 feet. These two vectors can be added together and the resultant can be drawn from the starting location to the final location. A sketch is shown below.

Since these displacement vectors are at right angles to each other, the magnitude of the resultant can be determined using the Pythagorean theorem. The work is shown below.
R2 = (44.9 ft)2 + (17.0 ft)2 = 2305 ft2
R = Sqrt(2305 ft2)
R = 48.0 feet
The angle theta in the diagram above can be found using the tangent function.
tangent(theta) = opposite/adjacent = (17.0 ft) / (44.9 ft)
tangent(theta) = 0.3786
theta = invtan(0.3786)
theta = 20.7 degrees
This is the angle which the resultant makes with the original line of motion (the 36.7 ft displacement vector).
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
58. A hiker hikes 12.4 km, south. The hiker then makes a turn towards the southeast and finishes at the final destination. The overall displacement of the two-legged trip is 19.7 km at 309 degrees . Determine the magnitude and direction of the second leg of the trip.
Answer: 12.7 km, 347 degrees
Like the previous problem (and most other problems in physics), this problem is best approached using a diagram.  The first displacement is due South and the resulting displacement (at 309 degrees) is somewhere in the fourth quadrant. (It is in the fourth quadrant because 309 degrees lies between 270 degrees or due South and 360 degrees or due East.) For communication sake, we will refer to the first displacement as A and the second displacement as B. Note that A + B = R. Since the magnitude and direction of the resultant is known, the x- and y-components can be determined using trigonometric functions. Since the angle of 309 degrees is expressed as a counterclockwise angle of rotation with due East, it can be used as the Theta in the equation.
The first displacement is due South and the resulting displacement (at 309 degrees) is somewhere in the fourth quadrant. (It is in the fourth quadrant because 309 degrees lies between 270 degrees or due South and 360 degrees or due East.) For communication sake, we will refer to the first displacement as A and the second displacement as B. Note that A + B = R. Since the magnitude and direction of the resultant is known, the x- and y-components can be determined using trigonometric functions. Since the angle of 309 degrees is expressed as a counterclockwise angle of rotation with due East, it can be used as the Theta in the equation.
Rx = R•cos(theta) = 19.7 km • cos(309 deg) = 12.398 km
Ry = R•sin(theta) = 19.7 km • sin(309 deg) = -15.310 km (the "-" means South)
Whatever the magnitude and direction of B is, it must add on to vector A in order to give a southward displacement of 15.310 km and a eastward displacement of 12.398 km. This could be expressed by mathematical equations as
Ax + Bx = 12.398 km, east
Ay + By = 15.310 km, south
But Ax is 0 km and Ay is 12 km, South. By substituting these two values into the above equations, the values for the x- and y-components of the unknown displacement can be determined:
Bx = 12.398 km, east and By = 2.910 km, south
 Knowing the Bx and By components will allow us to determine the magnitude and direction of B. Another diagram would help in visualizing the situation. The magnitude of B can be found using the Pythagorean theorem. B is the hypotenuse of a right triangle with legs of 12.398 km and 2.910 km. The sum of the squares of the sides is equal to the square of the hypotenuse.
Knowing the Bx and By components will allow us to determine the magnitude and direction of B. Another diagram would help in visualizing the situation. The magnitude of B can be found using the Pythagorean theorem. B is the hypotenuse of a right triangle with legs of 12.398 km and 2.910 km. The sum of the squares of the sides is equal to the square of the hypotenuse.
B2 = (12.398 km)2 = (2.910 km)2
B2 = 162.179 km2
B = Sqrt(162.179 km2)
B = 12.735 km
The direction of B is close to 360 degrees. As shown in the diagram, it is less than 360 degrees by an amount equal to theta. The angle theta can be determined using the tangent function and the length of the two sides of the right triangle.
Tangent (Theta) = (2.910 km) / (12.398 km)
Tangent (Theta) = 0.2347
Theta = Invtan (0.2347)
Theta = 13.2 degrees
The direction of B is 360 degrees - 13.2 degrees = ~347 degrees.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
59. A boat heads straight across a river which is 100. meters wide. For the following two combinations of boat velocities and current velocities, determine the resultant velocity, the time required to cross the river, and the distance traveled downstream.
|
a.
Given:
Boat velocity = 10.0 m/s, East
River velocity = 4.0 m/s, North
Calculate:
Resultant Vel. (mag. & dir'n): 11 m/s, 22 deg.
Time to cross river: 10.0 s
Distance traveled downstream: 40. m
|
b.
Given:
Boat velocity = 8.0 m/s, East
River velocity = 5.0 m/s, South
Calculate:
Resultant Vel. (mag. & dir'n): 9.4 m/s, 328 deg.
Time to cross river: 13 s
Distance traveled downstream: 63 m
|
Answer: See table above.
The two velocity vectors (boat and river) are directed perpendicular to each other. They can be added using the Pythagorean theorem. The direction is found using the tangent function; it is expressed as the counterclockwise angle of rotation from due East. The time to cross the river is dependent upon the width of the river and the boat velocity. And the distance downstream is dependent upon the time that the boat is moving and the speed at which it moves downstream - the river velocity.
|
a.
Resultant Vel. (mag. & dir'n):
R2 = (10.0 m/s)2 + (4.0 m/s)2
R = SQRT ( (10.0 m/s)2 + (4.0 m/s)2 )
R = SQRT(116 m2/s2) = 11 m/s (rounded from 10.8 m/s)
dir'n = invtan(4.0/10.0) = 22 degrees
Time to cross river:
d = v*t ---> t = d/v
t = (100. m)/(10.0 m/s) = 10.0 s
Distance traveled downstream:
d = v*t = (4.0 m/s)*(10.0 s) = 40. m
|
b.
Resultant Vel. (mag. & dir'n):
R2 = (8.0 m/s)2 + (5.0 m/s)2
R = SQRT ( (8.0 m/s)2 + (5.0 m/s)2 )
R = SQRT(89 m2/s2) = 9.4 m/s (rounded from 9.43 m/s)
dir'n = 360 deg. - invtan(5.0/8.0) = 328 degrees
Time to cross river:
d = v*t ---> t = d/v
t = (100. m)/(8.0 m/s) = 13 s (rounded from 12.5 s)
Distance traveled downstream:
d = v*t = (5.0 m/s)*(12.5 s) = ~63 m
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
60. The diagram at the right depicts a horizontally-launched projectile leaving a cliff of height y with a horizontal velocity (vix) and landing a distance x from the base of the cliff. Express your understanding of projectile kinematics by filling in the blanks in the table below. To simplify the calculations, use the approximated value for the acceleration of gravity value of -10 m/s/s.
|
|
vix
(m/s)
|
y
(m)
|
t
(s)
|
x
(m)
|
|
a.
|
15.0 m/s
|
20.0 m
|
2.00
|
30.0
|
|
b.
|
15.0 m/s
|
45.0
|
3.00 s
|
45.0
|
|
c.
|
15.0
|
45.0 m
|
3.00
|
45.0 m
|
|
d.
|
12.0
|
31.3
|
2.50 s
|
30.0 m
|
|
e.
|
17.2
|
74.0 m
|
3.85
|
66.0 m
|
|
Answer: See table above.
The solutions to all five of these projectile problems involve the use of kinematic equations and an appropriate problem-solving strategy. The kinematic equations and their use in projectile problems are listed and discussed elsewhere. The basic idea of the strategy is to identify three kinematic variables for either the horizontal motion or for the vertical motion. Once , three quantities in one direction is known, all other quantities in that direction can be found (or the time of flight can be found). Often, the time is then used with kinematic quantities for the second dimension in order to determine all other unknown quantities for that dimension.
In each of these problems, it is known that ax = 0 m/s/s, ay = -10 m/s/s, and viy = 0 m/s. When these three knowns are combined with the other given knowns the following answers are obtained:
|
|
Answers
|
Method
|
|
a.
|
t = 2.0 s and x = 30.0 m
|
Use y, viy, and ay to calculate t; then use t, vix and ax to calculate x.
|
|
b.
|
y = 45.0 m and x = 45.0 m
|
Use t, vix and ax to calculate x; and use t, viy, and ay to calculate y.
|
|
c.
|
vix = 15.0 m/s and t = 3.00 s
|
Use y, viy, and ay to calculate t; then use t, x and ax to calculate vix.
|
|
d.
|
vix = 12.0 m/s and y = 31.3 m
|
Use t, x and ax to calculate vix; and use t, viy, and ay to calculate y.
|
|
e.
|
vix = 17.2 m/s and t = 3.85 s
|
Use y, viy, and ay to calculate t; then use t, x and ax to calculate vix.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
61. The launch velocity and angle is given for three different projectiles. Use trigonometric functions to resolve the velocity vectors into horizontal and vertical velocity components. Then use kinematic equations to determine the time that the projectile is in the air, the height to which it travels (when it is at its peak), and the horizontal distance that it travels. (To simplify the calculations, use an acceleration of gravity value of -10 m/s/s.)
|
a.
Given:
Launch Vel. = 30.0 m/s
Launch angle = 30.0 degrees
|
b.
Given:
Launch Vel. = 30.0 m/s
Launch angle = 45.0 degrees
|
c.
Given:
Launch Vel. = 30.0 m/s
Launch angle = 50.0 degrees
|
|
Calculate:
vix = 26.0 m/s
viy = 15 m/s
tup = 1.5 s
ttotal = 3.0 s
y at peak = 11.3 m
x = 77.9 m
|
Calculate:
vix = 21.2 m/s
viy = 21.2 m/s
tup = 2.12 s
ttotal = 4.24 s
y at peak = 22.5 m
x = 89.9 m
|
Calculate:
vix = 19.3 m/s
viy = 23.0 m/s
tup = 2.35 s
ttotal = 4.69 s
y at peak = 26.9 m
x = 90.4 m
|
Answer: See table above.
The solutions to all three of these non-horizontally launched projectile problems involve the use of kinematic equations and an appropriate problem-solving strategy. The kinematic equations and their use in projectile problems are listed and discussed elsewhere. In each of these problems, it is known that ax = 0 m/s/s and ay = -10 m/s/s. The values of vix and viy can be determined using trigonometric functions:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
Once vix and viy are known, the other unknowns can be calculated. The time up to the peak (tup) can be determined using the equation
vfy = viy + ay *t
where the vfy = 0 m/s (there is no vertical velocity for a projectile when its at its peak) and ay = -10 m/s/s. Once tup is known, the ttotal (time to travel the entire trajectory -both up and down) can be determined by doubling the tup. The horizontal displacement of the projectile (x) can be computed in the usual way using the equation
x = vix*t + 0.5*ax*t2
where t is the ttotal value, ax = 0 m/s/s and vix was the first value calculated (using the trigonometric functions). Finally, the y at the peak (i.e., the peak height) can be calculated using the equation
y = viy*t + 0.5*ay*t2
where t is the tup value, ay = -10 m/s/s and viy was one of the first values calculated (using the trigonometric function). The t value in the equation is tup because the peak height is reached when the projectile has traveled for one-half of its total time; tup is that time. This method will yield the answers given in the table above.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
62. If a projectile is launched horizontally with a speed of 12.0 m/s from the top of a 24.6-meter high building. Determine the horizontal displacement of the projectile.
Answer: x = 27.0 m
This horizontally-launched projectile problem can be (and should be) solved in the same manner as the solution to #60 above. While #60 is broken down for you into nice steps, this problem is not so user-friendly. It is strongly recommended that you begin by listing known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = ???
vix = 12.0 m/s
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -24.6 m (- means moving down)
viy = 0.0 m/s (its launched horizontally)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time.
y = viy*t + 0.5*ay*t2
Plugging and chugging the above values into this equation yields a time of 2.25 seconds. Now the t value can be combined with the vix and ax value and used in an x-equation
x = vix*t + 0.5*ax*t2
to yield the answer 27.0 m. More examples and discussion of these types of projectile problems are discussed elsewhere.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
63. A projectile is launched with an initial speed of 21.8 m/s at an angle of 35.0-degrees above the horizontal.
(a) Determine the time of flight of the projectile.
(b) Determine the peak height of the projectile.
(c) Determine the horizontal displacement of the projectile.
Answer: (a) 2.55 s; (b) 7.98 m; (c) 45.7 m
This non-horizontally-launched projectile problem can be (and should be) solved in the same manner as the solution to #61 above. While #61 is broken down for you into nicely-structured steps, this problem is not so user-friendly. It is strongly recommended that you begin by resolving the initial velocity and angle into initial velocity components using the equations:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
This yields values of vix = 17.9 m/s and viy = 12.5 m/s. Once done, list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = ???
vix = 17.9 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = 0 m (it rises and falls to original height)
viy = 12.5 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there will be two solutions: t = 0 s and t = 2.55 s. These two solutions to the equation indicate that the time is 0 s when the vertical displacement (y) is 0 m. This is true before being launched (t = 0 s) and the instant it lands (t=2.55 s). The latter of the two solutions can be used to determine the horizontal displacement (x). Use the equation:
x = vix*t + 0.5*ax*t2
where t is 2.55 s, ax = 0 m/s/s and vix was the first value calculated (using the trigonometric functions). Plugging a chugging the above values into this equation yields the answer of 45.7 m.
Finding the vertical displacement at the peak (ypeak) demands using the original y equation with a time of 1.28 seconds (tup). This time corresponds to the time for one-half of the trajectory - the time at which the projectile will be at its highest or peak position. Substituting the viy, ay and t values into the equation
ypeak = viy*tup + 0.5*ay*tup2
yields a value of 7.98 m for the peak height.
More examples and discussion of these types of projectile problems are discussed elsewhere.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
64. A projectile is launched horizontally from the top of a 45.2-meter high cliff and lands a distance of 17.6 meters from the base of the cliff. Determine the magnitude of the launch velocity.
Answer: 5.79 m/s
The best means of starting this problem is to list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = 17.6 m (the distance horizontally from cliff base)
vix = ??? m/s
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -45.2 m (it falls down from the cliff to the ground)
viy = 0 m/s (it is horizontally launched)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there will be two solutions: t = 3.0372 s and t = -3.0372 s. A full parabola which follows the above function would have to locations where the y coordinate is -45.2 m. One would be "forward in time" at 3.0372 seconds; and the other solution is at a location traced "backwards in time" from the launch time. Of course, the positive answer is the one which we need; it can can be used to determine the initial horizontal velocity (vix). Use the equation:
x = vix*t + 0.5*ax*t2
where t is 3.0372 s, ax = 0 m/s/s and x = 17.6 m. Plugging and chugging the above values into this equation yields the answer of 5.7948 m/s.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
65. Two physics students stand on the top of their 3.29-meter second-story deck and launch a water balloon from a homemade winger. The balloon is launched upward at a speed of 45.2 m/s and an angle of 39.1 degrees. The balloon lands in a retention pond whose surface is 2.92 meters below grade. Determine the horizontal distance from launch location to landing location.
Answer: 211 m
This is a non-horizontally-launched projectile problem in which the initial velocity and launch angle are given. Three initial steps are always wisely taken before starting such a problem. First, determine the initial velocity components (vix and viy) using trigonometric functions. Second, construct a diagram of the physical situation. And third, organize known (and unknown) information in an "x-y table." These three steps are taken here. Quickly barging into a solution before giving the problem some pre-analysis often leads to a wasting of much time and ultimately a lot of confusion.
The initial velocity and angle can be resolved into initial velocity components using the equations:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
This yields values of vix = 35.077 m/s and viy = 28.507 m/s.
A diagram of the physical situation is shown.
Now the known values for each of the variables in the kinematic equations is listed in a table using a column for known horizontal information and a column for known vertical information.
|
Horizontal Motion
x = ???
vix = 35.077 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -5.49 m (from initial + to height to final - height)
viy = 28.507 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there will be two solutions: t = -0.1867 s and t = 6.004 s. A full parabola which follows the above function would have two locations where the y coordinate is -5.49 m. One location would be "forward in time" at 6.004 seconds; and the other solution is at a location traced "backwards in time" from the launch time. Of course, the positive answer is the one which we need; it can can be used to determine the horizontal displacement (x).
Now use the equation:
x = vix*t + 0.5*ax*t2
where t = 6.004 s, ax = 0 m/s/s and vix = 35.077 m/s (as originally calculated using the trigonometric functions). Plugging and chugging the above values into this equation yields the answer of 211 m. (Wow! Those boys had better be careful.)
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
66. A place kicker kicks a football from 39.6 meters from the goal posts. The kick leaves the ground with a speed of 24.8 m/s at an angle of 49.6 degrees. The goal posts are 3.10-meters high.
(a) Determine the amount by which the kick clears the goal posts.
(b) For this given launch velocity, what is the longest field goal (in yards) which could have been kicked? Assume that the football hits the horizontal crossbar of the posts and bounces through. Given: 1.00 meter = 3.28 feet.
Answer: (a) 13.7 m; (b) 64.7 yds (measured from kick location to goal posts)
(a) In part a of this problem, the task involves finding the height of the ball (y) when it has traveled a distance of 39.6 meters. The height of the goal posts can be subtracted from this value to determine the amount of clearance.
As is the case in all non-horizontally-launched projectile problems, it should be begun by resolving the initial velocity and angle into initial velocity components using the equations:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
This yields values of vix = 16.073 m/s and viy = 18.886 m/s. Once done, list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = 39.6 m (horiz. distance to goal posts)
vix = 16.073 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = ??? (we need to calculate this)
viy = 18.886 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of x-information are now known, an x-equation can be employed to find the time for the football to travel the horizontal distance to the goal posts. One useful equation is
x = vix*t + 0.5*ax*t2
in which case the time is 2.4637 s. The time can now be combined with a y-equations to find the vertical displacement (i.e., height above the ground) when the football has traveled horizontally to the goal posts. Use the equation:
y = viy*t + 0.5*ay*t2
where t = 2.4637 s, ay = -9.8 m/s/s and viy = 18.886 m/s. Plugging a chugging the above values into this equation yields the answer of 16.788 m.
When the ball has traveled a horizontal distance of 39.6 m, it is 16.8 m above the ground. The goal posts are 3.10 m high; so the ball clears the goal posts by 13.7 meters.
(b) Part b of this problem can be done in a similar manner. The task will involve first finding the time for the ball to rise to its peak and then fall back down to a height of 3.10 meters. Then the horizontal displacement can be calculated for this time. The final answer will then need to be converted to yards. The same vix and viy values can be used. Given the new context of the problem, the value for y is now known and x is unknown. The information can be organized in the usual x- and y- table.
|
Horizontal Motion
x = ???
vix = 16.073 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = 3.10 m (the height of the goal posts)
viy = 18.886 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there will be two solutions: t = 0.1718 s and t = 3.6825 s. The first solution corresponds to the first point along the parabola (during the rise of the football) when the football is at a height of 3.10 m and the second solution is the second point along the parabola (during the fall of the football) when the football is at a height of 3.10 m. The second answer can be used to determine the horizontal displacement (x) of the football. Use the equation:
x = vix*t + 0.5*ax*t2
where t = 3.6825 s, ax = 0 m/s/s and vix = 16.073 m/s. Plugging a chugging the above values into this equation yields the answer of x = 59.189 m. This x value can be converted to feet by multiplying by the 3.28 ft/m conversion ratio and then converted to yards by dividing by the 3.00 ft/yd conversion ratio. The kicker can kick as 64.7 yard field goal. (In football, it would be referred to as ~47 yard field goal since the goal posts are placed 10 yards behind the goal line and the ball is kicked from about 7 yards behind the line of scrimmage. Field goal distances are are measured from the goal line to the line of scrimmage.)
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
67. An airplane starts at Point A and flies 210. km at 311 degrees to Point B. The plane then flies 179 km at 109 degrees to Point C. Finally, the plane flies 228 km at 29 degrees to Point D. Determine the resulting displacement (magnitude and direction) from Points A to D.
Answer: R = 304 km, 24 degrees
Like most vector addition problems, this problem is best begun by the construction of a rough sketch of the physical situation. This sketch is shown below. There are three separate displacements taken by the airplane to result in a single displacement from point A to point D.
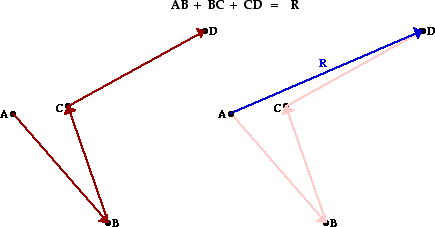
The actual solution is best performed using trigonometric functions to determine the x- and y-components of each displacement vector. These components are then added together to determine the x- and y-component of the resultant. The work is organized in a table below.
|
|
X-component
|
Y-Component
|
|
A to B
|
210. km • cos(311)
= 137.772 km, East
|
210 km • sin(311)
= 158.489 km, South
|
|
B to C
|
179 km • cos(109)
= 58.277 km, West
|
179 km • sin(109)
= 169.248 km, North
|
|
C to D
|
228 km • cos(29)
199.413 km, East
|
228 km • sin(29)
110.537 = km, North
|
|
Resultant
|
278.909 km, East
|
121.295 km, North
|
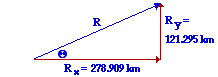
Once the components are known, the Pythagorean theorem can be used to determine the resultant. The resultant has components of 278.909 km, East and 121.295 km, North. The magnitude and direction of the resultant can be determined by adding these components. Since they are at right angles to each other, the magnitude can be determined using the Pythagorean theorem as shown below.
R2 = Rx2 + Ry2
R2 = (278.909 km)2 + (121.295 km)2 = 92502.800 km2
R = SQRT(92502.800 km2)
R = 304 km
The direction would be stated as the counterclockwise angle of rotation from due East. This is simply the angle Theta. Theta can be determined using the tangent function. The work is shown below.
Tangent(theta) = Ry/Rx
Tangent(theta) = (121.295 km) / (278.909 km) = 0.43489
Theta = Invtan(0.43489)
Theta = 23.5 degrees
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
68. Sammy Sosa clubs a homerun which sails 421 feet and lands on an apartment balcony located a vertical distance of 59.0 feet above the level of the ball-bat contact location. An observer times the flight to the balcony to take 3.40 seconds.
(a) Determine the velocity (magnitude and angle) at which the ball leaves the bat.
(b) Determine the speed of the ball (in miles/hour) when it lands in the bleachers.
Given: 1.00 m/s = 2.24 mi/hr; 1.00 meter = 3.28 feet.
Answer: (a) vi = 43.7 m/s at 30.2 degrees; (b) 88.3 mi/hr
(a) For any projectile problem, it always a wise idea to begin the solution with a listing of known and unknown information in an "x-y table." This is shown below.
|
Horizontal Motion
x = 421 ft = 128.35 m (horiz. distance to balcony)
vix = ???
ax = 0 m/s/s (true for all projectiles)
t = 3.40 s (the ball is a projectile for this long)
|
Vertical Motion
y = 59.0 ft = 17.99 m (vert. distance to balcony)
viy = ???
ay = -9.8 m/s/s (true for all projectiles)
t = 3.40 s (the ball is a projectile for this long)
|
Note that the time of flight is known. Time is a scalar quantity and has no directional component associated with it; one cannot refer to the horizontal time or the vertical time. It is listed in both tables since it can be used with kinematic equations for both the x- and the y-direction.
Since three pieces of x-information are known, an x-equation can be employed to find the initial horizontal velocity. One useful equation is
x = vix*t + 0.5*ax*t2
The initial horizontal velocity (vix) is 37.751 m/s.
There are also three pieces of y-information known. Thus, a y-equation can be used to determine the initial vertical velocity (viy). A good equation is
y = viy*t + 0.5*ay*t2
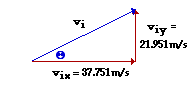 Plugging a chugging the above values into this equation yields an initial vertical velocity (viy) value of 21.951 m/s.
Plugging a chugging the above values into this equation yields an initial vertical velocity (viy) value of 21.951 m/s.
The ball leaves Sammy Sosa's bat moving upward with a speed of 21.951 m/s and moving horizontally with a speed of 37.751 m/s. These two components of the initial velocity can be used to determine the initial velocity and angle of the baseball after contact with the bat. A diagram is shown at the right. The initial velocity of the ball is represented by the hypotenuse of a right triangle with sides equal to the component values. Thus the Pythagorean theorem can be used to determine the initial velocity of the baseball.
vi 2 = (vix)2 + (viy) 2
vi2 = (37.751 m/s)2 + (21.951 m/s)2
vi 2 = 1906.97 m2/s2
vi = SQRT (1906.97 m2/s2 ) = 43.7 m/s
The angle (theta) of the initial velocity can be determined using a trigonometric function. The tangent function is used here.
Tangent(theta) = opposite / adjacent
Tangent(theta) = (21.951 m/s) / (37.751 m/s) = 0.58145
Theta = Invtan (0.58145) = 30.2 degrees
(b) In part (a) of this problem, the initial horizontal velocity was determined to be 37.751 m/s. For projectiles, this horizontal velocity does not change during the flight of the projectile. Thus, the projectile strikes the balcony moving with a final horizontal velocity (vfx) of 37.751 m/s. If the final vertical velocity (vfy) can be determined, then it can be used with the vfx value to determine the final velocity (vf). Several kinematic equations are useable for finding the final vertical velocity (vfy). The following equation will be used:
vfy = viy + ay•t
vfy = 21.951 m/s + (-9.8 m/s/s)•(3.4 s)
vfy = 21.951 m/s - 33.32 m/s
vfy = -11.369 m/s
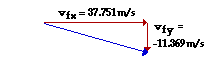
With the x- and y-components of the final velocity (vf) known, the Pythagorean theorem can be used to determine the final velocity value. A diagram is shown at the right and the calculations are shown below.
vf 2 = (vfx)2 + (vfy) 2
vf2 = (37.751 m/s)2 + (-11.369 m/s)2
vf 2 = 1554.41 m2/s2
vf = SQRT (1554.41 m2/s2) = 39.43 m/s
This value can be converted to miles/hour using the fact that 1.00 m/s = 2.24 mi/hr. The answer to part b is 88.3 mi/hr.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
69. An unfortunate accident occurred on the toll way. A driver accidentally passed through a faulty barricade on a bridge (quite unfortunately). and landed in a pile of hay (quite fortunately). Measurements at the accident scene reveal that the driver plunged a vertical distance of 8.26 meters. The car carried a horizontal distance of 42.1 meters from the location where it left the bridge. If the driver was in a 65 mi/hr speed zone, then determine the amount by which the driver was exceeding the speed limit at the time of the accident. Assume that the contact with the barricade did not slow the car down. (1.00 m/s = 2.24 mi/hr)
Answer: 72.6 mi/hr
This is an example of a horizontally-launched projectile problem. Like all projectile problems, the best means of starting the problem is to list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = 42.1 m (the horizontal distance which is traveled)
vix = ??? m/s
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -8.26 m (it falls down from the cliff to the ground)
viy = 0 m/s (it is horizontally launched)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there will be two solutions: t = 1.2983 s and t = -1.2983 s. A full parabola which follows the above function would have two locations where the y coordinate is -8.26 m. One would be "forward in time" at 1.2983 seconds; and the other solution is at a location traced "backwards in time" from the launch time. Of course, the positive answer is the one which we need; it can can be used to determine the initial horizontal velocity (vix). Use the equation:
x = vix*t + 0.5*ax*t2
where t = 1.2983 s, ax = 0 m/s/s and x = 42.1 m. Plugging a chugging the above values into this equation yields the answer of 32.426 m/s. This is the speed at which the car leaves the bridge at the start of its projectile motion. Converting this to mi/hr involves multiplying by the (2.24 mi/hr) / (1 m/s) conversion ratio. The result is 72.6 mi/hr.
(In reality, the car was traveling faster than this speed since the collision with the guard rail likely slowed the car down before it exited the bridge and began its projectile motion.)
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
70. Cupid wishes to shoot an arrow through the open window of a tall building. The window is 32.8 meters above the ground and Cupid stands 63.6 meters from the base of the building. If Cupid aims the arrow at an angle of 51.5 degrees above the horizontal, with what minimum speed must he fire the arrow in order for it to enter the window?
Answer: 32.7 m/s
Here is an example of a non-horizontally launched projectile problem in which the angle is given but the launch speed is not known. Thus, the x- and y- components of the initial velocity cannot be found. Nonetheless, expressions relating these components to the initial velocity can still be written and used in the problem.
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
The usual procedure of listing the known information in a "x-y table" is taken:
|
Horizontal Motion
x = 63.6 m (horizontal distance to building)
vix = vi * cos(51.5 deg) = 0.6225 • vi
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = 32.8 m (vertical distance from ground to window)
viy = vi * sin(51.5 deg) = 0.7826 • vi
ay = -9.8 m/s/s (true for all projectiles)
|
As shown in the table, there are only two pieces of x-information and two pieces of y-information given in the problem. Thus, there would seem at first to be insufficient information provided. But as is often the case in a real problem, one can forge ahead using variables in the hopes that there will be a means to introduce another equation which will assist in the solution. So both a horizontal and a vertical displacement equation will be written. (Note that units have been dropped form the solution in order to improve the clarity of the solution.)
|
Horizontal Displacement
x = vix*t + 0.5*ax*t2
 63.6 = (0.6225 • vi ) • t 63.6 = (0.6225 • vi ) • t
|
Vertical Displacement
y = viy*t + 0.5*ay*t2
 32.8 = (0.7826 • vi) •t + 0.5 • (-9.8) • t2 32.8 = (0.7826 • vi) •t + 0.5 • (-9.8) • t2
|
Now we have generated two equations with two unknowns and a solution can be found for the initial velocity of the arrow. Equation 1 is used to generate an expression for t in terms of vi. This expression is then substituted into equation 2 in order to solve for the initial velocity (vi). The work is shown below.
From equation 1: t = (63.6) / (0.6225 • vi )
Substituting into equation 2: 32.8 = (0.7826 • vi) •[(63.6) / (0.6225 • vi )] + 0.5 • (-9.8) • [(63.6) / (0.6225 • vi )]2
32.8 = (0.7826 • vi) •[(63.6) / (0.6225 • vi )] + 0.5 • (-9.8) • [(63.6) / (0.6225 • vi )]2
32.8 = 79.956 - 51145.94/(vi)2
-47.956 = -51145.94/(vi)2
(vi)2 = (-51145.94) / (-47.956)
(vi)2 = 1066.52
vi = 32.7 m/s
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
71. In a Physics demonstration, a projectile is launched from a height of 1.23 m above the ground with a speed of 10.6 m/s at an angle of 30.0 degrees above the horizontal.
(a) What horizontal distance from the launch location will the projectile land?
(b) With what speed does the projectile land?
Answer: (a) x = 11.7 m; (b) vf = 11.7 m/s
(a) As is the case in all non-horizontally-launched projectile problems, it should be begun by resolving the initial velocity (10.6 m/s) and angle (30.0 degrees) into initial velocity components using the equations:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
This yields values of vix = 9.180 m/s and viy = 5.30 m/s. Once done, list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = ??? (the unknown in part a)
vix = 9.180 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -1.23 m (vert. distance to floor)
viy = 5.30 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time for the projectile to rise and ultimately fall to the floor. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there are two solutions for the time: t = 1.2780 s and t = -0.1964 s. A full parabola which follows the above function would have two locations where the y coordinate is -1.23 m. One location would be "forward in time" at 1.2780 seconds; and the other solution is at a location traced "backwards in time" from the launch time. Of course, we wish to use the positive time value in our calculations. So t = 1.2780 seconds.
The time can now be combined with a x-equations to find the horizontal displacement (x). Use the equation:
x = vix*t + 0.5*ax*t2
where t = 1.2780 s, ax = 0 m/s/s and vix = 9.180 m/s. Plugging a chugging the above values into this equation yields the answer of 11.739 meters for the horizontal displacement.
(b) The landing speed (vf) of the projectile can be determined from values of the x- and y- component of the final velocity. Since the object being analyzed is a projectile, there is no horizontal acceleration and the final horizontal velocity (vfx) is the same as the initial horizontal velocity (vix) - 9.180 m/s. The final vertical velocity (vfx) can be determined using the following kinematic equation:
vfy = viy + ay•t
vfy = 5.3 m/s + (-9.8 m/s/s)•(1.2780 s)
vfy = -7.2244 m/s
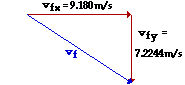
With the x- and y-components of the final velocity (vf) known, the Pythagorean theorem can be used to determine the final velocity value. A diagram is shown at the right and the calculations are shown below.
vf 2 = (vfx)2 + (vfy) 2
vf2 = (9.180 m/s)2 + (-7.2244 m/s)2
vf 2 = 136.462 m2/s2
vf = SQRT (136.462 m2/s2) = 11.7 m/s
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
72. A car is parked on a cliff overlooking the sea. The cliff is inclined at an angle of 29.0 degrees below the horizontal. The negligent driver leaves the car in neutral and it begins rolling from rest towards the cliff's edge with an acceleration 4.50 m/s/s. The car moves a linear distance of 57.2 m to the edge of the cliff before plunging into the ocean below. The cliff is 42.2 m above the sea.
(a) Find the speed (in m/s) of the car the moment it leaves the cliff.
(b) Find the time (in seconds) it takes the car to drop to the water below the edge of the cliff.
(c) Find the position (in meters) of the car relative to the base of the cliff when it lands in the sea.
Answer: (a) vf = 22.7 m/s; (b) t = 2.02 seconds; (c) x = 40.1 meters
(a) The first task involves using a kinematic equation to determine the speed of the car after accelerating from rest at 4.5 m/s/s for a distance of 57.2 m. The best equation is
vf2 = vi2 + 2•a•d
vf2 = (0 m/s)2 + 2•(4.5 m/s2)•(57.2 m) = 514.8 m2/s2
vf = SQRT(514.8 m2/s2) = 22.689 m/s
(b) Once the car reaches the edge of the cliff and rolls off, it becomes a projectile with a vertical acceleration of 0 m/s2. The second task involves determining the time of flight of the projectile from the cliff's edge to the water below. Like all non-horizontally launched projectiles, the starting point is to determine the initial horizontal velocity (vix) and the initial vertical velocity (viy). The initial velocity (22.7 m/s) and angle (-29.0 degrees) can be resolved into initial velocity components using the equations:
|
vix = vi * cos(theta)
|
viy = vi * sin(theta)
|
This yields values of vix = 19.844 m/s and viy = -10.999 m/s. Once done, list the known values for each of the variables in the kinematic equations. It is helpful to organize the information into two columns - a column of known horizontal information and a column of known vertical information.
|
Horizontal Motion
x = ???
vix = 19.844 m/s (from trig. function)
ax = 0 m/s/s (true for all projectiles)
|
Vertical Motion
y = -42.2 m (vert. distance to water)
viy = -10.999 m/s (from trig. function)
ay = -9.8 m/s/s (true for all projectiles)
|
Since three pieces of y-information are now known, a y-equation can be employed to find the time for the projectile to rise and ultimately fall to the floor. One useful equation is
y = viy*t + 0.5*ay*t2
in which case there are two solutions for the time: t = 2.0196 s and t = -4.2644 s. A full parabola which follows the above function would have two locations where the y coordinate is -42.2 m. One location would be "forward in time" at 2.0196 seconds; and the other solution is at a location traced "backwards in time" from the launch time. Of course, we wish to use the positive time value in our calculations. So t = 2.0196 seconds.
(c) The time can now be combined with a x-equations to find the horizontal displacement (x). Use the equation:
x = vix*t + 0.5*ax*t2
where t = 2.02 s, ax = 0 m/s/s and vix = 19.8 m/s. Plugging a chugging the above values into this equation yields the answer of 40.1 meters for the horizontal displacement.
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]
Navigate to:
Review Session Home - Topic Listing
Vectors and Projectiles - Home ||
Printable Version ||
Questions and Links
Answers to Questions:
All ||
#1-9 ||
#10-45 ||
#46-55 ||
#56-72
You Might Also Like ...
Users of The Review Session are often looking for learning resources that provide them with practice and review opportunities that include built-in feedback and instruction. If that is what you're looking for, then you might also like the following:
- The Calculator Pad
The Calculator Pad includes physics word problems organized by topic. Each problem is accompanied by a pop-up answer and an audio file that explains the details of how to approach and solve the problem. It's a perfect resource for those wishing to improve their problem-solving skills.
Visit: The Calculator Pad Home | Calculator Pad - Vectors and Projectiles
- Minds On Physics the App Series
Minds On Physics the App ("MOP the App") is a series of interactive questioning modules for the student that is serious about improving their conceptual understanding of physics. Each module of the series covers a different topic and is further broken down into sub-topics. A "MOP experience" will provide a learner with challenging questions, feedback, and question-specific help in the context of a game-like environment. It is available for phones, tablets, Chromebooks, and Macintosh computers. It's a perfect resource for those wishing to refine their conceptual reasoning abilities. Part 1 of the series includes Vectors and Projectiles.
Visit: MOP the App Home || MOP the App - Part 1